When will it warm-up in Fairbanks? Adjusting Climatology for Trend

Hi, Rick T. here with some ideas on how we can use the historical record (i.e. climatology) in the face of significant trends. What I want to look at in this post are estimating threshold exceedance dates, e.g. first or last date in the season of the temperature exceeding some threshold. This is very commonly available for freeze dates, such as this plot of the probability of first date of freezing temperature at Fairbanks Airport in autumn (courtesy of the Western Regional Climate Center), but of course can be constructed for any threshold of interest. However, an underlying assumption for the use of this kind of information for planning or forecasting is that the past is a reliable guide to the future, i.e. there are no significant trends. 

The high temperature so far (through April 27) at Fairbanks Airport has been 54F. This is only slightly lower than average for the historical record, but is notably low for recent years: only four springs in the past 30 years have had a lower high temperatures at this point in the season. So when is going to really get warm? Or at least, when can we expect to get warm based on the usual annual cycle? We of course turn to climatology. The first thing we can do is construct a plot like the one above just based on the historical data. From this, we see that the median date of the first 70F is May 21, and any day between May 15 and May 25 would be pretty typical, while dates before May 7th or June 1st would be quite unusual.

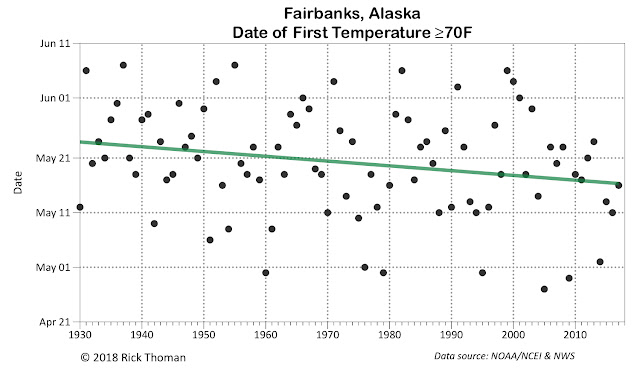
However, when we plot the first 70F of the warm season as a time series, we see that the the first occurrence of 70F has been occurring, on average, earlier in recent years, as the the linear regression trend line slopes downward (and is statistically significant at the 95% confidence level), with the typical first 70F now about week earlier than in the 1930s.

So how could we adjust our cumulative distribution plot to account for the trend? If we assume that the variablity remains the same, and that looks like a reasonable assumtion in this case, then it's pretty easy:

• First detrend the observed dates by, for each year, subtracting the regression value from the observed. This leaves us with the regression residuals. 
• Next, take the regression estimate for the present (in this case May 16th) and add that back into the residuals. This in effect "converts" the historical record to the current climatology.
• Last, construct a cumulative distribution plot on our "converted" data. 

When we do that, it makes a significant difference because of the trend:
So based on our present climate, we would estimate that there is about a 30% chance of having the first 70F temperature in Fairbanks before May 12th, whereas using only the historical record we would estimated less than a 20% chance. Similarly, the chances of getting through May without having a 70F or higher temperature is now less than 5%, half of the historical-only estimate. Is any of this meaningful? That of course depends on your needs.

Putting it all together, here is the final graphic, with the cumulation distibution plots for 60F, 70F and 80F temperatures adjusted to reflect the probablies of exceedance based on today's reality and not on a different past.